| Lowell 1 | Peirce MS 472 (SPIN) | Arisbe | Peirce pages on this site |
transcribed and edited by Gary Fuhrman, May-June 2018, from the manuscript copies made available by the SPIN project (link above). In a few places punctuation has been added for clarity. Parts of this lecture have been previously published in CP 6.88-97 and NEM3.
Lowell Lectures 1903
Sixth Lecture. Probability.
Ladies and Gentlemen:
More than once during this course I have declared that I do not now touch upon metaphysics, but confine myself to logic. I do not mean that what I have said has no bearing upon metaphysics. On the contrary, metaphysics can properly draw its principles from no other source than logic. It ought to consist in the interpretation of the facts of common experience in the light of a scientific logic. That is the method of Plato, of Aristotle, and of Kant. Logic is the prior science. Its truths ought to be worked out without squinting at all at their metaphysical bearings. No man with a natural genius for logic who devotes his life to the study of it will ever tolerate the smallest interference from metaphysics, any more than a geologist would tolerate any interference from theology.
But in taking up the confusing question of chance, I know that your minds are wedded to vague metaphysical doctrines about it, which are rife the world over, especially among men who pride themselves upon thinking clearly; and I do not think I would bring your thoughts fully to bear on the pure logical question without a few introductory words on the metaphysics of chance, especially since some of you are probably aware that my own opinions about this subject are what might be described as extreme.
CP 6 numbering given from here on, but the text follows the manuscript.
88. The question what are the shares of uniformity and variety in the phenomena of the universe, is a question which has never been agitated in any public dispute that has attracted general attention. The consequence is that everybody in a semi-unconscious way forms his own opinion about it, usually a pretty vague opinion; and he has the impression that all his neighbors think as he does about it. But, on questioning people closely, it will be found that there are no less than five different opinions that are widely spread on this subject. I will briefly state them and point out just how much arbitrariness each supposes.
89. Beginning with the middling one, in the degree of arbitrariness that it allows, which is, no doubt, the opinion of the largest party among those who know enough of dynamics to entertain such a conception, this view is that the material universe is composed of particles of some kind, each having at any one instant its position in space, and also its velocity, determinate in direction and in amount; and it is held that physical laws are of such a nature that according to the positions of the particles at any instant their velocities are, at that instant, changing at perfectly determinate rates and in perfectly determinate directions. This party holds that, if this statement were rendered definite by indicating just what these accelerations are in each position of the particles, it would be the perfect résumé of all the laws of nature. As for consciousness, these persons hold that its states are rigidly dependent upon the instantaneous states of the physical universe, and that it need not be taken into account in saying what will happen in that universe.
This theory makes the uniformity to be perfectly exact and inflexible. It is such that, given the positions and velocities of all the particles at any one instant, the positions and velocities at all other instants are precisely determined and with these the exact phenomena of all consciousness and feeling. But it supposes the positions and velocities of all the particles at one instant to be entirely arbitrary. It further regards the law itself, although as a law it is general, [as] yet arbitrary in respect to what its requirements are.
90. If we designate the five classes of minds who entertain the five opinions by the first five letters of the alphabet, the A's being the persons who admit the least arbitrariness and the E's being those who admit the most, then the opinion just formulated is that of the C's. If you should be out walking on a fine starlit night in company with a B and a C, and were to point up to the heavens and ask your companions whether they supposed there was any law determining the arrangement of the stars, C would smile and would remark that not a single law of that description had ever been discovered yet. Thereupon B would exclaim, “What! Do you mean to say there is no regularity in the arrangement of the stars when there is the Milky Way before your eyes!” “Oh,” C would reply, “there is a rough and irregular compression of the cluster in which we happen to find ourselves. But, assuming the Nebular Hypothesis to be true, that could hardly escape coming about in consequence of the cluster being very much condensed from a former state in which its velocity happened to be a little greater on one side than on the other, which constituted a rotation. But however it came about, the fact that the arrangement is so excessively rough shows at once that it is due to some accident and not to law, since the effects of law are rigidly exact.” To this B might perhaps reply, “I do not think your explanation very satisfactory. You suppose that a manifest regularity of arrangement among millions of stars is the effect of an accident. It seems much easier to suppose that the regularity of arrangement was once perfect, but that the motions of the separate stars have deranged it.” While this dialogue has been going on an adherent of the sect of A's has joined the group. He now says, “But you surely will admit that if the original perfect symmetry of arrangement has been broken up, probably in its passage into some different form of symmetry, the present apparently irregular arrangement must have been fully intended by the Creator.” B replies, “I do not quite know that I am prepared to admit that the world ever was created. But even if it was, while the positive intentions of the Creator must have been fulfilled, we need not suppose that he expressly intended every relation between facts. If the Dowager Empress of China happens to have a fit of coughing and just at that moment I, on the other side of the globe, happen to take a piece of hoarhound candy, we need not suppose that this coincidence was any part of the Creator's plan.” A replies, “I believe that Providence overrules every fact and relation however trivial; and even if I were in your state of scepticism, I should still hold it to be inconceivable that any state of facts should fail to conform to some law. You cannot shuffle a pack of cards so that there is no mathematically exact relation between the arrangement before shuffling and the arrangement after shuffling.”
So there you have the three commonest forms of necessitarianism. A holds that every feature of all facts conforms to some law. B holds that the law fully determines every fact, but thinks that some relations of facts are accidental. C holds that uniformity within its jurisdiction is perfect, but confines its application to certain elements of phenomena.
91. The party of the D's, of which I am myself a member, holds that uniformities are never absolutely exact, so that the variety of the universe is forever increasing. At the same time we hold that even these departures from law are subject to a certain law of probability, and that in the present state of the universe they are far too small to be detected by our observations. We adopt this hypothesis as the only possible escape from making the laws of nature monstrous arbitrary elements. We wish to make the laws themselves subject to law. For that purpose that law of laws must be a law capable of developing itself. Now the only conceivable law of which that is true is an evolutionary law. We therefore suppose that all law is the result of evolution, and to suppose this is to suppose it to be imperfect.
92. Finally, there are those who suppose nature to be subject to freaks, who believe in miracles not simply as manifestations of superhuman power but as downright violations of the laws of nature, absolutely abnormal. Professor Newcomb, for example, in a series of articles which he contributed to the Independent, suggests that the human will has a power of deflecting the motions of particles, in plain violation of the third law of motion. I do not think, by the way, that it is generally known that some of the early Fathers of the Church refused to believe in physical miracles; and apparently attributed them to a superhuman hypnotic power, reminding one of what the Hindoo jugglers have made British officers think they saw. St. Augustine, on the contrary, while holding it impious to think them to be violations of Nature's Laws, regards them apparently as occurrences that are to us what the reading of a letter by a man might seem to a dog to be, namely, a manifestation of some higher mastery of things than would be compatible with his nature.
93. One fallacy into which the necessitarians of class C generally fall is that they imagine that they can disprove that anything happens by chance by showing that the event has a cause. Thus Boëthius, at the beginning of the fifth book of his consolations, after citing Aristotle as a necessitarian, which is enough to take one's breath away, so monstrous is the blunder or the impudence of it, has a little ode of twelve lines which Mr. Henry Rosher James translates in 24, that imitate the swing of the original very well, but miss the point. By a geographical fiction Boëthius represents that the Tigris and the Euphrates flow from a common lake. Now suppose a boat to be wrecked in that lake and one part of it is carried down the Tigris, the other part down the Euphrates, and where these rivers, after being separate for hundreds of miles, flow together again those two parts of the boats are dashed against one another. There is a fortuitous event if there ever was one; and yet, says Boëthius, the currents forced them to move just as they did so that there was no chance about it. True, the existential events were governed by law. But when we speak of chance, it is a question of cause. Now it is the ineluctable blunder of a nominalist, as Boëthius was, to talk of the cause of an event. But it is not an existential event that has a cause. It is the fact, which is the reference of the event to a general relation, that has a cause. The event, it is true, was governed by the law of the current. But the fact which we are considering is that the two pieces that were dashed together had long before belonged together. That is a fact that would not happen once in ten thousand times, although when you join to this fact various circumstances of the actual event, and so contemplate quite another fact, it would happen every time, no doubt. That is to say, nobody can doubt it but an adherent of the E's sect. The example is a very good one as showing that the causal necessitation of more concrete fact does not prevent a more prescinded, or general, fact of the same event from being quite fortuitous. The position of Aristotle in this matter is altogether right, and not “veri propinqua ratione,” as Boëthius says; but it is a position that nobody can understand who is completely [immersed] in the state of mind of modern philosophy. Zeller, for example, does not seize it at all.
94. But let us drop metaphysics and return to logic. It was Hobbes who first said, referring to and combating Aristotle's doctrine, “Men commonly call that casual whereof they do not perceive the necessary cause,” for Hobbes was a typical stoic in his philosophy. Leibnitz emphatically agrees with Hobbes. “Fort bien,” he says. “J'y consens, si l'on entend parler d'un hasard réel. Car la fortune et le hasard ne sont que des apparences, qui viennent de l'ignorance des causes, ou de l'abstraction qu'on en fait." This has been said a thousand times since with an air as if it explained the whole thing. I do not doubt that that is the impression of almost everybody in this hall. But I am quite sure that most of you will be glad to reëxamine the question with me. I will just give you the headings of some thoughts about it, which, if it is not too great a liberty, I would suggest that you take note of and carefully pursue by yourselves when you find leisure. I wish in the latter part of this lecture to make some remarks of great importance in many reasonings; and in order to get any time for those remarks I shall be obliged to make my statement of this part so brief that only the most thorough student of philosophy could fully grasp the meaning of it at the single hearing.
95. The first thing to be taken into consideration is the general upshot of Kant's Critic of the Pure Reason. The first step of Kant's thought,— the first moment of it, if you like that phraseology,— is to recognize that all our knowledge is, and forever must be, relative to human experience and to the nature of the human mind. That conception being well digested, the second moment of the reasoning becomes evident, namely, that as soon as it has been shown concerning any conception that it is essentially involved in the very forms of logic or other forms of knowing, from that moment there can no longer be any rational hesitation about fully accepting that conception as valid for the universe of our possible experience. To repeat an example I have given before, you look at an object and say “That is red.” I ask you how you prove that. You tell me you see it. Yes, you see something; but you do not see that it is red; because that it is red is a proposition; and you do not see a proposition. What you see is an image and has no resemblance to a proposition, and there is no logic in saying that your proposition is proved by the image. For a proposition can only be logically based on a premiss and a premiss is a proposition. To this you very properly reply, with Kant's aid, that my objections allege what is perfectly true, but that instead of showing that you have no right to say the thing is red they conclusively prove that you are logically justified in doing so. At this point, the idealist appears before the tribunal of your reason with the suggestion that since these metaphysical conceptions, that repose upon their being involved in the forms of logic, are only valid for experience and since all our knowledge is relative to the human mind, they are not valid for things as they objectively are; and since the conception of existence is preeminently a conception of that description, it is a mere fairy tale to say that outward objects exist, the only objects of possible experience being our own ideas. Hereupon comes the third moment of Kant's thought, which was only made prominent in the Second Edition, not, as Kant truly says, that it was not already in the book, but that it was an idea in which Kant's mind was so completely immersed that he failed to see the necessity of making an explicit statement of it, until Fichte misinterpreted him. It is really a most luminous and central element of Kant's thought. I may say that it is the very sun round which all the rest revolves. This third moment consists in the flat denial that the metaphysical conceptions do not apply to things in themselves. Kant never said that. What he said is that these conceptions do not apply beyond the limits of possible experience. But we have direct experience of things in themselves. Nothing can be more completely false than that we can experience only our own ideas. That is indeed without exaggeration the very epitome of all falsity. Our knowledge of things in themselves is entirely relative, it is true; but all experience and all knowledge is knowledge of that which is, independently of being represented. Even lies invariably contain this much truth, that they represent themselves to be referring to something whose mode of being is independent of its being represented. This is true even if the proposition relates to an object of representation as such. At the same time, no proposition can relate, or even thoroughly pretend to relate, to any object otherwise than as that object is represented. These things are utterly unintelligible as long as your thoughts are mere dreams. But as soon as you take into account that Secondness that jabs you perpetually in the ribs, you become awake to their truth. Duns Scotus and Kant are the great assertors of this doctrine, for which Thomas Reid deserves some credit, too. But Kant failed to work out all the consequences of this third moment of thought and considerable retractions are called for, accordingly, from some of the positions of his Transcendental Dialectic. Nor in other respects must it be supposed that I assent to everything either in Scotus or in Kant. We all commit our blunders.
96. To this first consideration, it is necessary to add, in the second place, that of the great difference in the logical status of the future and the past, which Aristotle stated with great emphasis without finding anybody in modern times to comprehend what he said, not even Trendelenburg, who comes the nearest to it. Aristotle is understood by modern critics to be in a childishly naïve state of mind on this subject. Now it is quite true that Aristotle was almost the first pioneer in logic and just stood at its threshold. It is also true that there are some monumental follies in his physical books; but the worst of these may fairly be presumed to be insertions made by different students during the thirty years when his manuscripts lay on the shelves of his school for general use. But Aristotle was by many lengths the greatest intellect that human history has to show; and it was precisely in such fields of thought, as this distinction of past and future time, that his mind was the most thoroughly trained. So gigantic is his power of thought that those critics may almost be excused who hold it to be impossible that all of the books that have come down to us as his should all have been produced by one man. I am ashamed to have to confess that I shared the general opinion of Aristotle's childish naïveté in those passages, until the further progress of my own studies forced me to the very substance of what Aristotle says. The past is ended and done; the future is endless and can never have been done. To be sure, if we regard past time as having had no beginning, then, when we make general assertions concerning it, we can only be talking of it as an object of possible experience, that is, of what future researches may bring to light. Hence it might be inferred that the contrast Aristotle speaks of between the past and the future might be merely subjective, having to do with our different attitude toward them. But even a moderate appreciation of the Kantian argument will show that, besides being true in regard to our knowledge of time, it must also be regarded as true of real time; and time is real, whether we accept Kant's dubious view of it, which he is certainly far from making evident, as the form of the internal sense, or not. I do not question Time's being a form, that is, being of the nature of a Law, and not an Existence; nor its being an Intuition, that is, being at the same time a single object; nor its having a special connection with the internal world. But I doubt very much whether Kant has succeeded in rightly stating the connection between those three features of Time.
97. Now there are three characters which mark the universe of our experience in a way of their own. They are Variety, Uniformity, and the passage of Variety into Uniformity. By the Passage of Variety into Uniformity, I mean that variety upon being multiplied almost in every department of experience shows a tendency to form habits. These habits produce statistical uniformities. When the number of instances entering into the statistics are small compared with the degree of their variation, the law will be extremely rough, but when the number runs up into the trillions, that is to say cubes of millions, or much higher, as in the case of molecules, there are no departures from the law that our senses can take cognizance of.
CP 6.97 ends here.
You will find one such rough uniformity illustrated in two maps in ‘Studies in Logic by Members of the Johns Hopkins University.’ Here is another that I have dug out of our last Census on purpose for this lecture. The comparison is of the number of deaths per thousand of the population in the years 1890 and 1899 respectively, in over a dozen countries the comparison being made with numbers calculated from the law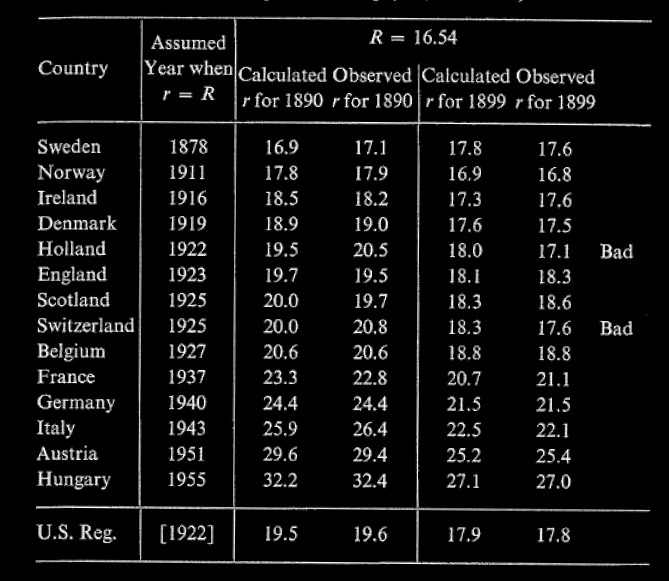 [table in the manuscript]
[table in the manuscript]
The best way of settling the meaning of a word is to take it in such a sense as shall render the word most useful. Now chance is the foundation of the great business of Insurance; and the doctrine of Chances has been without much exaggeration called the logic of the exact sciences. But when chance is said to consist in our ignorance, it certainly can be of no use except to those who desire to prey upon us. The Insurance business is not run on ignorance in any further sense than that if a man knew when he was going to die he would not insure his life. That which renders chance so important is that there is immense diversity throughout the universe. Diversity in many respects with uniformity in a few respects, and a great tendency among diversities to grow into uniformities are three real objective characteristics of the universe.
I will now drop metaphysics and consider the doctrine of chances. In order that this doctrine should have any useful application, it is necessary that we should positively know a number of propositions to be true. The matter by no means rests on mere ignorance. Of course, the doctrine of chances supposes a certain amount of ignorance, since it is a method of attaining knowledge; and every method of attaining knowledge must suppose that the information it teaches us how to obtain is not already in our possession. But there must be some large class of objects, say the As, which have already presented themselves in experience and of which we have reason to believe that many other instances will present themselves in future experience. Now the As of which we have had experience have some of them had a certain quality, while others of them have been without this quality. I shall say some As are Q and some are not Q, using Q as an adjective expressive of that quality. Since we have experienced only a finite collection of As, it must be that there has been some definite proportion of those As we have experienced that have been Q and we must have some good reason for supposing that this same ratio or some ratio to which it approximates is going to hold in regard to our future experiences of As. Or rather, what it is that we must know,— not with absolute certainty, for such knowledge is impossible as to future experience, but still what we must know, in such sense as the future can be known,— what we must know is not that our individual future experience will show the same ratio between the number of As that are Q and the number of those that are not;— in case we have that kind of knowledge, which very rarely happens, the problem is specialized so as to become quite unlike the ordinary problem of chances. But what we must know is that, supposing the state of things does not undergo any change that makes any material difference in the result, that same ratio would hold good in the long run. A sufficiently clear understanding of the doctrine of chances to make it safe to apply it to any novel case requires an accurate conception of what a long run is. We shall soon have to give our closest attention to this matter. But it will be best to begin by taking note of all the elements of the problem, so that we may know what are the nice points that require analysis. We must not [only] have a sufficient knowledge that this ratio is going to continue substantially unchanged, but we must further know that there is not going to be any law of succession according to which As that are Q and As that are not Q are going to present themselves. If for example every other A is going to be Q and every other one not Q, the laws of chance will not hold. It is the same with any other law of succession. We must not only not know that [there] is such a law, but must have a sufficient assurance that there is no such law. The books on the subject are full of the word “independent.” The instances must be independent. We must make sure that they are so. This “independence” that is so much insisted upon is nothing but the absence of any law of recurrence. All the good writers insist that we must assure ourselves of the independence of the “events,” as they call the instances; so that they teach that it is not enough to be ignorant of law, but that it [is] requisite that we should make sure that there is no law of the kind that is pertinent to the question. Now information of this description does not involve any kind of knowledge of future individual instances. There may be in such knowledge ground for an inference as to individual instances; but the doctrine of chances institutes no sound inquiry into such inferences. Some writers on the subject endeavor to judge of such inferences by the principles of the calculus of probabilities; but what they say on the subject is, as I can clearly demonstrate, utterly worthless; and being so, insofar as it is apt to be accepted as sound, it is most mischievous. If a lady is afraid of going on board a steamer, it is a good argument to say to her that only one passage in thousands is accompanied by any serious harm to a passenger, and that she therefore ought not to hesitate to embark. But to say to her that the doctrine of chances teaches what it is wise to do in an individual case is a serious error of logic. Such inference is of a kind concerning which the doctrine of chances affords no direct knowledge. All that the doctrine of chances can do is to say what will happen in the long run. For example, suppose a pair of dice to be thrown. Call them die M, and die N. Now we know that die M will turn up an ace once in six times, or six times out of thirty-six, in the long run, and that die N will not only in the long run turn up a deuce once in six times, but will do so in the long run once in six of those six times out [of] thirty-six in which die M turns up an ace. Therefore, once in thirty-six times in the long run M will turn up an ace and N a deuce, and by parity of reasoning, once in thirty-six times M will turn up a deuce and N an ace; so that deuce-ace will in the long run be thrown twice in thirty-six or once in eighteen times.
We do not know, however, that this will happen in any finite succession of throws. Therefore, by a long run we must understand an endless run. But there is no such thing as a half, or a third, or any other definite finite proportion of the members of a denumeral collection. For instance, you might be tempted to say that one whole number out of every three is divisible by 3. But all whole numbers can be arranged in this way
1 3 2 6 (3) 9 4 12 5 15 (6) 18 7 21 8 24
You will evidently thus get all the whole numbers. Yet 3 out of every 5 are divisible by 3.
Or they may be arranged in two series so as to follow in different proportions in the two sequences
2 3 6 11 12 15 20 21 24 29 30 33 . . .
1 4 5 7 8 9 10 13 14 16 17 18 19 22 24 26 27 28 29
A finite ratio of a denumeral collection only acquires a meaning when that collection has a fixed serial order. That amounts to saying that chance only relates to the order of experience or order of existential succession. What is called “geometrical probability” is not properly probability at all, unless it receives specifications not germane to the real problem, so as to put it into the guise of probability. Chance, then, in the sense in which the doctrine of chances studies it, consists in a statistical law and no other law governing the succession of a species of events in the endless future.
Generally, in all its meanings, chance refers to variety, in contradistinction to uniformity. We call a fact accidental, if it is not governed by a specified or well-understood general formula or other general idea, such as a general intention. But it would be an excellent practice to restrict the expression “happening by chance” to meaning happening so in a series of experiences, which series must be distinctly specified to give the phrase any meaning, that the fact is not governed by any order of succession that holds in the long run, no matter whether it be intended, or otherwise necessitated, or not.
The manuscript includes at this point a section that Peirce says “will have to be skipped”, in a note on his p. 92. This section is omitted here but included in the transcription on the SPIN site beginning at page 50.
When we say that the frequency with which the As are Q, or in other words the probability that an A will be Q, has a given value, p, what we mean is, that in any denumeral succession of occurrences of As taken in the order in which they occur in the course of experience, if we take any finite range of conceivable values that includes p … if, I say, whatever such range of values may be taken, there will come a time in the succession of the As after which the proportion of As that are Q among all the As from the beginning never ceases to be included within that range, then, and only then p is the frequency with which the As are Q in the long run, or in other words, is the probability that an A will be Q. Of course, we cannot say at any time when that time will be or has been after which the value of the ratio in the evergrowing tally wiil never leave that range of values. For if we could do so, that would constitute a law in the succession and the occurrences would not be independent. But nevertheless the time will come, supposing the occurrences are independent. There must be some ultimate frequency; for otherwise there would be a law of alternation of some kind in the succession.
I now come to the most important part of this lecture. The calculus of probabilities proceeds entirely by mathematical reasoning, that is to say by necessary reasoning. Such inferences as it draws are necessary inferences. If it sounds incongruous to speak of necessary inferences of probability, this is perhaps merely an apparent incongruity not a real one. But if it is a real incongruity it is because the word probability ought not to be applied to ratios of frequency which are no more subjective or modal, in their nature than any other statistical averages. The use of the word probability for the average frequency in the long run of experience is a fault of a much graver nature than ill-chosen expressions usually are. It is a fault similar to that of measuring
energy in “foot-pounds,” which since a pound is a pound and a foot is a foot all the world over leads people to forget that a footpound in Edinburgh and a footpound say in the Philippines differ considerably; but the fault of the word probability is worse than that inasmuch as the errors it leads to cannot be considered as trifling, however rough a measurement may satisfy us. We speak of the probability of an event. It is not the event but the fact that ought to be spoken of, a fact being an element of an event resulting from an act of mental discrimination. However, that is, comparatively speaking, a trifle. When we so speak we only mention one term of the ratio. What would you think if I were to talk to [you] about
the ratio of a house, as if a ratio were some absolute quality of the house? You would say that in the first place, it is not the house, but some dimension or measure of the house that can have a ratio. That is a fault similar to speaking of the probability of an event instead of the probability of a fact, or of the truth of a proposition. But this you would say is a small absurdity compared to that of speaking of the ratio of a thing without mentioning but one term of the ratio. If anything has a ratio, it must be a ratio to something. A proposition may have a probability without any second member, provided the term probability be used in another and perhaps more legitimate sense.
But in the sense in which probabilities can be evaluated and made the subjects of mathematical calculation, a probability is a ratio of frequency in the long run of experience. This should never for one instant be lost sight of; and you should constantly ask yourself ‘Is there going to be any such experience in any way that can give the particular probability spoken of any real utility?’ The majority of treatises on probability tell us that if a man who never heard of the tides were to wander to some shore where the tides are very noticeable and were to notice that the tide rose in each one [of] any number of successive half days, say N for N half days, then the probability that it would rise on the next
half day would be (N+1)/(N+2). But what is the long run of experience that this represents? The tide it is said would be found to rise the next half day in N + I cases out of every N + 2 taken at random. But what are these N + 2 cases, I ask. They will say, I suppose, that they are the cases in which I observe a new phenomenon on N successive occasions. But it is impossible to suppose any solid statistics with such vague conditions. It all depends on what sort of facts one would call a new phenomenon. I go out with a friend for a walk in the country. We successively meet three acquaintances and my companion remarks that all three were baptists.
Has this any necessary bearing on what the communion of the next man we meet may be? Mind, I grant at once
that some kind of argument might be founded upon it. But we are talking of arguments capable of being deduced mathematically; for the calculus of probabilities confines itself to mathematical reasoning. Two things are possible, either that we meet successively four baptists or that we meet three baptists first and then a non-baptist. Now if these events are independent, which is the only case in which the calculus can be applied at all, then representing by b the probability of a man met on that road being a baptist the probabilities of the two cases that are
now the only remaining possibilities were at the beginning of our walk
b4/(b3 (1-b) + b4) = b/((1-b) + b) = b
That is, the probability is just what it was in the first place. If the events are not independent still the probability of the fourth man being a baptist must be greater the greater the antecedent probability of it. Yet the real argument that he will be a baptist has no force except from the fact that it is a strange thing to meet three baptists successively. But of course, to say that the events are independent, precisely amounts to saying that you cannot argue from one to another.
If you carefully examine the line of thought of these writers, you will find that they assume throughout, that we must at all times be in a condition to know what the probability of a future occurrence is. Now if probability is to be anything but a delusion it must have some objective meaning. To know a probability must be to know something. To know that the probability of a coin turning up heads is ½ must be to know, in a positive (I do not say in an infallible) sense that it will turn up heads half the time in the course of our future experience. Yet Laplace, the master of those writers, founds his whole theory on the express assumption that if we know nothing about a coin or even as he remarks if we know it is loaded one way or the other the probability that it will turn up heads is ½, so long as we do not know which way it is loaded. This is the πρῶτον ψεῦδος of their whole argument. You note that in the formula (N+1)/(N+2) if N is zero, that is if the new phenomenon has never been observed at all, its probability is ½. Mr. F. Y. Edgworth, who is a thinker of no inconsiderable ability (notwithstanding his somewhat puerile delight in airing his contempt for my writings), regards it as an important basis for the probabilities, with the business of insurance and all that, that of just one half of all possible questions answerable by yes or no, “yes” is the true answer. To talk of extracting gold from sunbeams after that sounds rather flat.
The only security against utter nonsense in this subject is to give up talking of probabilities in connection with the doctrine of chances and to talk instead of ratios of frequency in the course of our future pertinent experience. I insert the word “pertinent” to indicate, for example, that an insurance company does not want to know what death-rate they may learn of from the returns of the board of health but what the death-rate is going to be among the people they insure. I do not care what proportion of possible questions can truly be answered by “yes”; but if I could know what proportion of my theories of logic were going to withstand criticism that information would be very welcome.
Eminent mathematicians have proposed to apply the calculus of probabilities to determining judicial decisions, and to express the “veracity” of a witness by a number equal to the proportion of questions that he will answer truly.
You remember the story in Rabelais of the judge who acquired such a great reputation for the eminent fairness and impartiality of his decisions that the King sent for him to explain his method. Whereupon he explained that his rule was first carefully to read the arguments on both sides and then take a pair of dice and give judgment for one or the other party according as the throw was odd or even.
There is one further feature of probability or unordered frequency to which I must draw your attention. It is quite obvious that a fact may happen independently with any frequency expressible by a rational fraction. It is less obvious that the ultimate frequency can have an irrational value without there being any law of succession; but there seems to be no doubt that this also is possible. For although it is impossible that the ratio of frequency should, at any stage of the endless succession of occurrences, be other than a vulgar fraction, yet given any two values, one greater and tbe other less than the irrational value, there is no reason why as the succession proceeds the ratio for all that part of it that has occurred should not at length forever cease to be so great as the greater of the two values while ceasing to be as small as the smaller one. Thus, that question seems to be satisfactorily answered.
A point which often puzzles those who are entirely ignorant of the doctrine of chances is that they think that if a coin has turned up heads say ten times in succession,— which will happen about once in a thousand times,— since it must turn up heads and tails in equal proportions, the long run of heads must be followed by throws among which there is an extra proportion of tails, to balance the extra heads. This of course is a great mistake. The extra heads though they were a million would not affect the relative frequency of heads in the long run, in the slightest degree. Everybody who has studied the doctrine of chances knows this, though perhaps not everyone understands it quite as clearly as he fancies he does, for not a few are puzzled by what is precisely the same thing, namely, the fact that it is not logically impossible that an event whose probability is zero should nevertheless occur on millions of occasions.
For example, three men, A, B, and C, agree to play a perfectly even game on the following conditions. Only two can play at a time, playing against each other; while the third stands by to take the place of the first one who goes out. At any one play one player wins a dollar from the other and the chances are perfectly even. The dollar is not actually paid over until one of the players retires temporarily from the table, and no player does retire until he has netted a gain during the sitting. Now the doctrine of chances shows that at a perfectly even game the probability that a player whose cash and credit amount to c dollars, will net a gain of n dollars before he is ruined will be c/c+n. At this game the credit of the players is unlimited, and thus the probability will be infinity divided by infinity plus one, which fraction is strictly equal to 1. That is, the probability that a player will net a gain is 1, or certainty. Thus the three go on playing, each one as soon as he has made his absolutely certain net gain of a dollar, yielding his seat to the third; and so they keep on for all eternity, every man winning bis dollar at every sitting, which dollar is paid in solid cash though the credit is unlimited while the sitting lasts.
I have known persons to find little conundrums similar to this quite puzzling.
Hume applies the doctrine of chances to disprove miracles. Now a miracle would not be a miracle if it were not entirely out of the ordinary course of nature, or what would be the course of
nature to a materialist. As such the probability of a miracle is zero. But that does not logically conflict with its happening millions of times. It is logically possible that a die should turn up a six; and therefore it is logically possible that it should turn up an endless succession of sixes and never any other side, although the probability of it is zero. But that a die should turn up a seven is logically impossible. That would be more than a miracle. It would be an absurdity.